theorytore.blogg.se - Cube flip and turn worksheet

CUBE FLIP AND TURN WORKSHEET HOW TO

Your fingers will move in the same way the object will move. The right-hand rule works as follows: point your thumb parallel to the axis you are rotating about and curve your fingers naturally towards the palm of your hand.

The right-hand rule is often used in physics and math to calculate vectors and rotations of objects. Now we need to define the difference between a positive and a negative rotation with the right-hand rule (show slide 24).

Can you find the rotation of the gray object that is analogous to the rotation of the white object?Ĭopyright © 2016 Jacob Segil, College of Engineering and Applied Science, University of Colorado Boulder We’ll start out rotating about one-axis.įigure 1. Objects can be rotated about the three axes-x, y and z-and any combination of the three. In this activity, we are going to rotate both isometric drawings and blocks-very similarly to what you did on the Spatial Visualization Practice Quiz at the beginning of the associated lesson. For example, how do you know which direction to put a key in a lock? How do you organize your locker so that you can fit in more books? These tasks require the ability to visualize the one-dimensional rotations of objects. We use object rotation to understand the world around us-what objects are and how they fit together.

(Have the slide presentation up and displayed to the class, starting with slide 21.) Today we are going to learn about rotations! Rotating objects is an essential skill in the engineering world and it’s something we most likely already do every day.

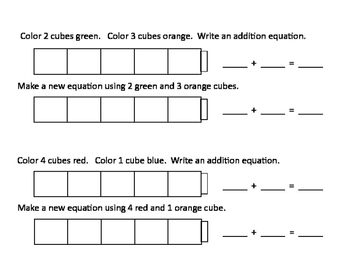
CUBE FLIP AND TURN WORKSHEET HOW TO

Use the right-hand rule to explore rotations of objects.īefore taking part in this spatial visualization activity, students should have taken the Spatial Visualization Practice Quiz and learned about spatial visualization in the associated lesson, Let’s Learn about Spatial Viz! They should know about isometric drawing, how to use triangle-dot paper and coded plans (as can be learned in the associated activity, Connect the Dots: Isometric Drawing and Coded Plans), as well as orthographic views (as can be learned in the associated activity, Seeing All Sides: Orthographic Views).Students should be familiar with the Cartesian coordinate system (x-, y-, z-axes) and degrees of rotation.

Translate the rotation of one block to a second block.

Spatial visualization is an essential skill for engineers to be able to take ideas to the next level that is-ideas that initially only exist in the mind to something that can be communicated clearly to other engineers, and eventually turned into products, structures and systems.Īfter this activity, students should be able to: Engineers use one-axis rotation drawings to better visualize 3-D objects to make sure their ideas represented on 2-D screens and paper work in the real (3-D) world in which the designs, products and inventions must function and operate. Rotating objects is a technique used in spatial visualization and is part of understanding and effectively utilizing engineering software. To use a texture fill, click Texture, and then click the texture that you want to use.Students studying architectural plans during a spatial visualization project period. To use a gradient effect for the selected fill color, click Gradient, and then click the gradient style that you want to use.įor additional gradient styles, click More Gradients, and then in the Format Chart Area pane, under Fill, click the gradient options that you want to use. In the Insert Picture dialog box, click the picture that you want to use, and then click Insert. To fill the shape with a picture, click Picture.

In the Colors dialog box, use the available options to pick a color, and then click OK. To use a fill color that is not available under Theme Colors or Standard Colors, click More Fill Colors. To remove the color from the selected chart element, click No Fill. To use a different fill color, under Theme Colors or Standard Colors, click the color that you want to use. Click the Shape Format tab, and click ( Shape Fill icon).